Ask someone his age and he will tell you. Ask how he knows and the reply takes more thought. Usually it is on the authority of his parents, who told him his birth date. With the age of the Earth the situation is rather different. Although geologists may assume the role of authority figures, they were not present when the Earth came into being, and the planet has no birth certificate. To come up with an age requires a dating system and a dating theory, and if the theory is to be trusted, it needs to be tested against the thing being dated. A coroner may perform an autopsy and deduce that the corpse is of a woman in her 70s, but if the unwrinkled face suggests a girl in her teens, we should suspect there is something wrong with the dating method. Common sense plays a role.
For a rock to be datable, something in it must have changed over time at a regular rate. This is what radioactive elements have done. They emit particles and thereby decay into other elements. Though we cannot predict exactly when an atom will emit a particle, the decay takes place at a statistically constant rate, and if we can deduce what the parent-daughter ratio was at the time the rock crystallised, the present ratio enables us to calculate how much time has elapsed since. Obviously enough, the method applies only to rocks that contain radioactive decay products.
It also applies only if the rate of decay has always been the same. Suppose the rate had decreased over time, gradually tailing off to zero. As the currently measured decay rate (or half-life) of an element has no theoretical basis and could in the past have been any value, the only way to test whether present rates apply is to compare the results against the direct evidence of passing time. They need a ‘reality check’, much as the conclusion of our imaginary coroner needed a reality check. Unfortunately, this is never done. If a geological sequence appears to have built up more rapidly than present rates suggest, the dating system is left unquestioned. The sequence is assumed to include long intervals which left no geological record and the theory thereby protected from being falsified.
Geology comes closest to verifying its timescale when comparisons are made with the time taken for the Earth to complete certain astronomical cycles. Although somewhat speculative, the idea is that the cycles may generate long-term swings in climate strong enough to be expressed in sedimentary sequences. If a sequence has a pattern consistent with the repetition of astronomical cycles, it may be possible to calculate its entire length. The approach is similar to tree-ring dating. Working back from the present, the aim is to link such sequences into one master sequence, and compare the astronomical timescale with the one based on isotope decay.
The shortest astronomical cycles are the day, month, and year. In the case of the year, the cycle is defined by the seasons. Since the Earth’s axis is at an angle relative to its orbit round the Sun, a hemisphere experiences summer when it is tilted towards the Sun and winter when it is tilted away. The seasons constitute the primary astronomical cycle. Three others affect the intensity of the seasons.
The Earth’s tilt, its obliquity, is currently 23.5 degrees. The greater the tilt, the greater the difference between summer and winter. Over the time that accurate measurements have been made the tilt has decreased at a rate of 1.19 metres per century. The tilt is thought to oscillate between a minimum of around 22 degrees and a maximum of 24.5 degrees. 
A complete cycle is calculated to take 41,000 years, with the period very slowly getting longer.
The shape of the Earth’s orbit round the Sun also varies, from a near-perfect circle to an ellipse. This pattern of change, called the eccentricity cycle, extends over 98,000 years. When the orbit is markedly elliptical, summers which coincide with the shortest distance between the Sun and the Earth are warmer than those which coincide with the longest distance.
Finally, there is the phenomenon known as precession, where the elliptical orbit of the Earth turns around itself, and the Earth’s axis wobbles around itself, like the axis of a spinning top. Precession of the Earth’s orbit increases the seasonal contrast in one hemisphere while decreasing it in the other. At this present stage in the cycle the Earth is closest to the Sun when the northern hemisphere is in winter, so northern winters are less cold and summers less warm. Precession of the Earth’s axis (thought to be caused by the pull of the Moon and the Sun on the equatorial bulge) causes a corresponding shift in the celestial poles. Today the North Star is in the constellation Ursa Minor, but 5,000 years ago the North Star was in the constellation Draco. The precession cycle varies between 19,000 and 23,000 years, again, slowly increasing with time.
The three cycles of precession, obliquity and eccentricity are known as Milankovitch cycles, after the scientist who proposed that these long-term variations have a noticeable effect on climate. Milutin Milankovitch published his theory in 1941, but it was not until the 1980s that geologists began applying it to sedimentary sequences, for example chalk. Where deposits were stratified in couplets and sedimentation seemed to have been fairly continuous, one couplet could often be interpreted as indicating a warm climate and the other a cool climate.
Chalk consists predominantly of microscopic plates from warm-water algae known as coccolithophores. Although from a distance it can appear a monotonous mass of white or grey, it is in fact bedded. In some places, beds of relatively pure chalk alternate with beds of marl, chalk mixed with clay. In others, compact and densely burrowed beds alternate with ones that are not so compact or densely burrowed. Often the tops of beds are marked by nodules of flint.
The couplets are interpreted to reflect the precession cycle, with the chalk accumulating when the climate was warmer and drier and the marl when the climate was colder and wetter. Clay eroded from the land more quickly when the weather was rainy, thereby diluting the chalk. Although one may question whether the precession cycle should cause ‘wetter’ to go with ‘cooler’ and ‘drier’ with ‘warmer’ (the reverse would also be possible) oscillations in oxygen isotope ratios (δ18O) appear to confirm the interpretation. The oscillations reflect changes in temperature and they trace a broadly similar pattern. Climate change thus provides an explanation for the occurrence of bedding.
If the Milankovitch theory is correct, such cyclicity can be used to test radioisotope dating. For example, if the system attributes 3 million years to a continuous sequence and the precession cycle was 20,000 years, the sequence should comprise 150 such couplets. Substantially more or fewer than 150 would be evidence that the timescale was in error. In practice, the two chronological systems rarely give the same result. Sometimes approx- imate agreement is achieved only on the premise that the couplets reflect obliquity rather than precession, or a mixture. Some studies claim to have detected cyclicity corresponding to all three Milankovitch cycles. In such instances the smallest cycle is visible as repeating couplets and the larger cycles as ‘bundles’ of couplets, a bundle of four or five being seen as evidence of the eccentricity cycle. Thus, in relation to the Biancone Formation, in the Alps, Mayer and Appel (1999) report:
Cycle periods of 45 cm, 80 cm and 180 cm likely correspond to dominant precession, obliquity and eccentricity cycles. Owing to the inaccuracy of the Cretaceous time scale, periods cannot be matched exactly, but cycle ratios are extremely close to expected ratios.
Since the two chronological systems do not agree, and the timescale based on radioisotope dating is not as precise as outsiders tend to assume, it is the latter which is judged inaccurate. Milankovitch cycles offer higher resolution, down to 20,000 years instead of uncertainties of a million years or more, and geochronologists have recently been using them to ‘tune’ their timescale. The latest internationally agreed timescale (Ogg et al. 2016) tunes radioisotope dates back to the Miocene. Portions of the Triassic, Jurassic, Cretaceous and Palaeogene have also been redated by this method.
Astronomical tuning is a rash move, for if the only criterion for determining whether a sedimentary sequence reflects Milankovitch cycles is the degree of closeness to the isotope timescale, there is no independent control on either method. The Milankovitch interpretation is upheld by producing a result approximately consistent with the radioisotope dates, and the radioisotope timescale then adjusted to it. The reasoning is circular.
If the danger is not clear enough from the Biancone Formation, it is well illustrated by the debate over a succession in the western Dolomites, where 470 metres encompass around 600 bedding cycles. After making allowance for variable rates of sedimentation, researchers thought they could detect signals relating to both precession and eccentricity. According to Preto and Hinnov (2003):
All of the principal periodicities related to the precession index and eccentricity… are present: P1 (21.9 ky); P2 (17.8 ky); E1 (400 ky), E2 (95 ky), and E3 (125 ky), along with a peak at a frequency double that of the precession, which is a predicted feature of orbitally forced insolation at the equator. Components possibly related to Earth’s obliquity at ca. 35 ky and ca. 46 ky are present as well.
(P1 and P2 designate the different precession cycles, E1-E3 the different eccentricity cycles, ‘ky’ is a thousand years.) This Triassic succession preserved ‘the oldest pristine Milankovitch signature yet observed in the geologic record’. Other workers rejected the interpretation, finding that radioisotope ages from interspersed volcanic-ash layers indicated a much shorter interval. The procedures involved in analysing cyclical bedding could generate Milankovitch-like periodicities (once calibrated to the isotope timescale) that were nothing of the sort: the appearance of a close fit with the astronomical cycles was illusory.
There may also be mathematical grounds for thinking so. According to Mensur Omerbashich (2006), the pro- cedures which generate claims that bedding cycles reflect multiple kinds of astronomical cycle are questionable. In the case he analysed, the alleged 99% confidence level was ‘mostly meaningless in the context of total-information quality’, owing to prior editing of the data and failure to distinguish between signal and noise. Another critical study (Vaughan et al. 2011) was able to validate only one of four previously published claims of Milankovitch periodicity. Because many cycles were spurious, the vast majority of cycle detections derived from stratigraphic data were likely to be false.
Discrepancies between the two timescales, some minor, some major, can be documented many times over (e.g. Hilgen & Langereis 1989, Karner & Muller 2000, cf Ehrlich 2007). In order to save the theory, it has even been suggested that obliquity can dominate in one locality and precession, over the same interval, in another (Prokoph et al 2001, Krijgsman et al 2004, Nederbragt et al 2007). In short, like radioisotope dating, Milankovitch theory is an example of a scientific paradigm (Kuhn 1962), an all-embracing explanation which provides the framework within which ‘normal science’ can operate but which is rarely itself tested or questioned. Problems are identified and solved, so far as possible, within its terms. Anomalies with the potential to undermine the paradigm are ignored, ascribed to errors in the data, or accommodated by modifying the paradigm, while faith in the paradigm remains strong. Thus, science can be both critically questioning in the domain where normal science takes place and unquestioning at the level where what is at stake is its world-view and a sense of belonging to a brotherhood of common values and beliefs.
That said, the phenomenon of cyclical bedding is real, and if it does not reflect Milankovitch cycles, it must reflect some other rhythm. To return to the example of chalk, beds are often very extensive. In one case they have been traced from outcrop to outcrop over more than one and half thousand miles (Gale et al 1999). Explanations are required that apply on a global scale.
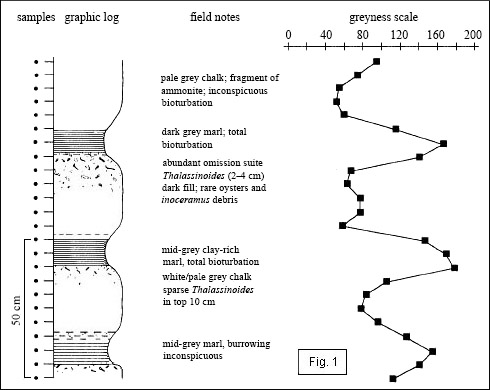
What if the warm-cool cycle of each couplet was of summer and winter, the prime astronomical cycle, rather than precession, so that we were looking at the deposit of just one year? Figure 1 (from Gale et al) illustrates a series of couplets from the Cenomanian part of the Upper Cretaceous. The grey-scale fluctuations track the proportion of clay in the chalk, from 5-15% in the light-coloured beds to 10-30% in the darker beds. The top of each cycle is an omission surface, where sedimentation ceased for a time and animals made burrows. The marls are totally bioturbated, suggesting that they provided a more oxygenated or nutrient-rich environment for deposit-feeders.
Dense bioturbation does not take thousands of years. Experiments have shown that a small population of deposit-feeders, such as the crustaceans that made the Thalassinoides-type burrows (Fig. 1), can churn a 10 cm depth of sediment in 42 days (Gingras et al. 2008). Since the chalk and marl beds are not homogenised, this is the kind of timescale that should be considered when making interpretations.
Another ‘remarkable’ observation – remarkable if the couplets represent large, long-term shifts in climate – is the failure to show any long-term shifts in faunal composition (Lauridsen et al. 2009). While the fauna in the marl is more diverse than in the chalk, their mode of life remains the same, and no species are restricted to just one rock type. They were well adapted to both environments. The marked change in water temperature through the course of these cycles had no effect.
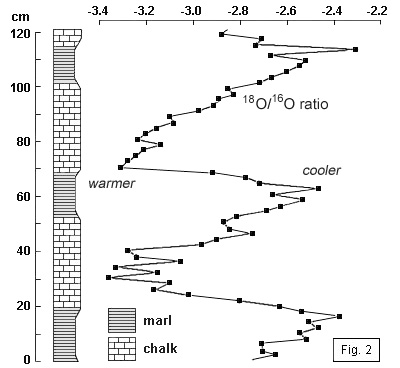
Figure 2 shows the δ18O profile of three chalk-marl couplets in the ‘white cliffs’ between Dover and Folkestone, also of Cenomanian age. The zig-zag pattern follows that of the greyness scale (Fig. 1), and since low oxygen isotope ratios in sea-floor oozes correlate with warm sea-surface temperatures, and vice versa, explaining this as the effect of summer and winter does not seem unreasonable. The same pattern may be seen in the shells of individual organisms. For example, a study of the tropical bottom-dwelling foram Cyclobirculina, which has a life span of one year, recorded a variation in δ18O ratio from -0.5 in the summer to +1.5 in the winter (Wefer & Berger 1980 and Fig. 3). Growth rate increased with age, peaking in the spring. Similar patterns have been detected in other kinds of carbonate-secreting organism, both from the past (Purton & Brasier, 1999) and from the present (e.g. Dunbar & Wellington 1981, Hickson et al. 1999).
Since coccolithophores are much smaller than forams and have a life span of only days or weeks, the ooze composed of their remains would reflect the annual oscillation collectively, not individually. They are more prevalent in the chalk than the marl and are thought to represent productivity blooms (Leary et al. 1989). The chalks are dominated by microplankton that lived in the top 100 metres, the marls by microplankton that lived in deep water or on the sea bottom. Temperatures rose steeply in the course of marl deposition, peaked near the base of the chalk beds, then fell less steeply (Fig. 2). Fossil escape burrows have been reported from some bases (Leary et al. 1989), indicating that the rate of deposition in the lower part of the chalk was sometimes extremely high. This would also account for the relatively sharp transitions from marl to chalk. The compositional difference would be due to higher rates of planktonic productivity during spring and summer.
In modern settings the rate of coccolith flux to the seafloor has also been observed to oscillate annually. Around the Canary Islands, sedimentation peaks in February/March following phytoplankton blooms in the winter (Sprengel et al. 2002). In the Arabian Sea the settling rate peaks in January and declines to very low levels in August through to November (Andruleit et al. 2004). Thus the hiatus in sedimentation at the top of the chalk beds is directly comparable to the virtual cessation of coccolith flux from late summer to late autumn in modern seas.
Similar rhythms characterise the chalks that formed towards the end of the Cretaceous, during the Maastrichtian. As with outcrops onshore, cores extracted from the North Sea show metre-scale cycles, but here the alternation is between compact, intensively burrowed chalk and porous, mostly unburrowed chalk (Scholle et al. 1998); in the lower part of the cycle the sediment retains its primary laminar structure. There is little bioturbation other than the escape shafts of animals momentarily buried by dumps of sedimentary snow. Along with the high porosity this indicates a rapid rate of sedimentation. Further up, the rate slowed, allowing burrowers to destroy the laminae. Calcite cementation began to harden the seafloor, enhancing burrow preservation, but insufficient time elapsed prior to the resumption of heavy sedimentation for hardgrounds to form.
In the Central Graben of the North Sea the chalk cycles consist of bioturbated beds and slightly thinner non-bioturbated, mainly laminated beds (Damholt & Surlyk 2004). The laminae alternate between graded layers thought to have been deposited from small-volume turbidity currents and suspension clouds and non-graded layers deposited from a rain of pelleted coccoliths directly from the plankton. In either case, only the briefest of timescales is required, minutes in the case of the graded laminae, hours in the case of the ungraded ones. The bioturbated beds imply longer timescales, perhaps a few months.
According to conventional dating, the Cretaceous period lasted 80 million years – a stretch of time that, accustomed though we are to such figures, is unimaginable. Since the figure is based on data extraneous to the properties of the rocks themselves, those properties can provide a test of the timescale, and as we have seen, in the case of chalk sequences they do not agree with ages of this magnitude. If we consider the evidence on its own merits, we have no grounds for inferring the miniscule rates of sedimentation imposed by the radioisotope timescale.
A typical chalk-marl couplet has a thickness of around 40 cm. Before compaction the thickness may have been double that, around 80 cm. Averaged over 20,000 years, this gives a sedimentation rate of 0.04 mm, or half a hair’s breadth, per year. That too is difficult to imagine. By contrast, if the couplets were annual, the sedimentation rate would have averaged 2.2 mm per day – higher during productivity blooms (sufficient, as we have seen, to bury organisms alive), lower at other times.
Measurements of modern sedimentation rates tend to be expressed in milligrams per square centimetre. With solid chalk having a density of around 2,500 mg (2.5 g) per cc, the uncompacted density would be about 1,250 mg per cc. Thus, if chalk-marl couplets represented 20,000 years, the sedimentation rate would be 5 mg per year or 0.014 mg per day; if the couplets represented one year, the rate would be 100 g per year, or 274 mg per day. Modern rates vary according to the same factors that ancient rates vary, but are closer to 5 mg than to 100 g per year. Broerse et al (2000) reported a carbonate flux of 2.2 mg per square centimetre per year, which is even lower than that calculated for the Cenomanian.
However, modern rates do not necessarily provide the right comparison. In normal conditions, low productivity and low settling velocity result in high rates of consumption by animal grazers and high rates of chemical dissolution. In bloom conditions, the rate of production increases by orders of magnitude and faecal-pellet clumping leads to greatly increased settling velocity, with consequently low rates of consumption and low rates of dissolution (Wal et al. 1995). Coccolithophores, it should be noted, are ‘the most productive calcifying organisms on earth’ and can reach a density during blooms of some 115 million per litre. Chalk formations bear witness to the exceptional ‘hypercalcifying activity of the coccolithophorids’ (Aubry 2005).
The main factors governing the dissolution of calcium carbonate are temperature, acidity, water depth and saturation state. Calcium carbonate dissolves more readily where the water is colder, more acidic, deeper and less saturated. In the inland seas where the Cretaceous chalks were laid down the conditions for calcium carbonate precipitation were close to optimal. The seas were warm (the global climate itself was exceptionally warm), not acidic, and shallow, and they had high concentrations of the ions that make up calcium carbonate: Ca2+ and CO32-.
Another point about the Cretaceous is that the ratio of magnesium to calcium in seawater was much lower than in modern seas. High levels of Mg have been found to inhibit growth rates. In experiments involving three species of coccolithophore in low-Mg seawater all showed large increases in population growth rates; two of the species reproduced at rates approximately triple those observed in modern seawater (Stanley et al 2005). The species dominating Cenomanian chalks are unlikely to have been less responsive. The ubiquitous Watznaueria barnesae, for example, is thought to have been highly prolific (Lees et al 2005). Although the data are less clear, the same seems to have been true of Biscutum constans .
- The Chalk consists almost entirely of the remains of coccolithophores, which in the right conditions can reproduce at prodigious rates.
- The warmth and shallowness of the waters where the coccoliths accumulated meant that a much smaller percentage of platelets shed by the coccolithophores dissolved before they reached the bottom.
- The low Mg/Ca ratio of Cretaceous seawater and the high absolute concentration of calcium favoured coccolithophore reproduction.
- Some of the coccolithophore species that dominated chalk assemblages are known to have been prolific examples of their kind.
Coccolithophore blooms can occur only if there is a sufficient supply of inorganic nutrients, calcium ions and dissolved carbonate. Once the supply is exhausted the blooms cease, and they will not have the potential to recur until the minerals are replenished. Thus in the context of sedimentation rates of around 1 metre per year the final question to consider is how the minerals could be replenished that quickly.
Seawater chemistry is controlled primarily by the flux of hydrothermal brine from mid-ocean ridges. Cretaceous seas were rich in some elements, including Ca2+ and CO32-, because rates of ocean crust production were higher (Hardie 1996).
On the assumption of unchanging rates of radioactive decay, the rate of ocean crust production in the Middle Cretaceous is estimated to have been almost twice the modern rate, thereafter declining to present-day values. The seafloor spreading rates are nonetheless tiny, of the order of centimetres per year. On the other hand, if radioactive decay was higher in the past, the increased heat output would have sped up all geological processes, including volcanism at mid-ocean ridges. Large amounts of CO2 would have been introduced into the ocean from underwater volcanism and large amounts of calcium from the hydrothermal alteration of ocean crust. The calcium carbonate removed by coccolithophore blooms would thus have been quickly replenished. Along with levels of atmospheric CO2 ten times higher than now (Royer et al 2004), the whole phenomenon of chalk may therefore be seen as indirect evidence of elevated levels of plate-tectonic activity.
Absolute dates in geology are based on radioactive decay, where the key assumption is that the rate of decay has been constant over time. These dates command acceptance because they accord with a world-view in which long ages are required. The idea of subjecting them to critical testing is so heretical that it is not even entertained privately, let alone discussed in the literature.
Although Milankovitch cycles have the potential to test radioisotope dating, they come with their own presuppositions – concerning the solar system’s age, the imperturbability of the Earth-Moon system over time and the ability of astronomical cycles to dominate sedimentary processes. They are part of a world-view which assumes the constancy of isotopic half-lives, and in practice they are used only to ‘tune’ the geological timescale, not test it. Discrepancies between the two chronologies are minimised in a variety of ways, including interpreting some cycles as relating to precession, others to obliquity, and postulating that some cycles are not preserved. In reality, ‘very few rhythmically bedded geological sections yield clear-cut power spectra when calculated against stratigraphic depth, whatever the age of the section’ (Nederbragt et al. 2007).
As well as being self-consistent, isotope dating needs to be consistent with the nature of what is being dated. Our contention is that it is fundamentally inconsistent. This website sets out several instances where it conflicts with direct indications of passing time (for links, see the Key Concepts section). Here we have focused on the possibility that the warm-cool cycles seen in relatively tranquil offshore settings during the Cretaceous were annual, and therefore represent sedimentation rates orders of magnitude higher than those inferred from the radiometric timescale. Perhaps the most obvious evidence is the pattern of bioturbation through chalk beds. Given sedimentation rates of 0.04 mm per year, mobile deposit feeders should have repeatedly ploughed through their entire depth; no part should have been left undisturbed. In fact, bioturbation is limited.
Assuming that cycles attributed to precession (the shortest Milankovitch period) were annual, a simplistic calculation would divide the 80 million radioisotope years of the Cretaceous by 20,000 to arrive at a true duration of around 4,000 years. However, Milankovitch cycles do not necessarily provide a good fit even for sections that are well dated, and the appearance of chronological precision can be illusory. Estimates of the duration of Ocean Anoxic Event II (latest Cenomanian), for example, vary from 320,000 years to three times that figure. Short intervals are chronologically more difficult to quantify than long intervals.
As one approaches the historical period, the disparity between radioisotope and true time decreases. Consequently the rhythmicity that continues to be visible in sedimentary successions far into the Cenozoic may be expected to be even less amenable to Milankovitch explanations.
Chalk beds and time images
